
Problem solving is a key component of DISCOVER, forming a basis for both the Assessment and the Curriculum Models. But the DISCOVER approach to problem solving is different. It is built upon the premise that considering a problem’s structure and method (how it is solved) is as important as actually finding the solution. Each is briefly summarized below:
Problem Structure—The way a problem is formed and presented is critical to its effect on learning. An effective use of problem solving must include problems that vary widely. DISCOVER rates Problem Structure on a scale that ranges from what we call a “Type I” problem, up to a “Type V” problem. A Type I problem is highly structured and closed whereas a Type VI problem is completely unknown and needs to be created. All conceivable problems fall somewhere in the continuum between the two extremes. For sake of illustration, we break the continuum down into Problem Type I, Type II, Type III, Type IV, Type V, and Type VI.
Problem Method—The way a problem is solved will depend on its form and presentation, as discussed above. A Type I problem can be solved in only one way and the solver simply must know the right method, to arrive at the right solution. A Type VI problem, on the other hand, is unkown for the presenter and the solver, and it might have an infinite number of ways to arrive at a solution. The solver must determine which method(s) might be better than others and if any given method is even appropriate…with the possibility that there are no appropriate methods because there is no definitive solution. Consideration of Problem Method is an important part of developing critical thinking skills.
Problem Solution—The Type I problem has a single right answer (ex. 1+1=2). A pure Type VI problem is so abstract that it might have an infinite number of possible solutions or it might not have a solution at all. Arriving at a “right” answer for a Type VI problem is usually highly subjective, depending on what method was chosen and on how the initial problem was created and interpreted in the first place (ex. “Does God exist?”).
Figure 1 below illustrates what we call the “DISCOVER Problem Continuum” (Maker and Schiever, 1991), a tool used in assessment and curriculum. The six Problem Types are displayed, along with how much information is known—how much structure is provided—for both the problem presenter and the problem solver in each Problem Type. A detailed analysis follows below. The DISCOVER Problem Continuum actually is an expanded version of a model developed by Getzels and Csikszentmihalyi (1976,1967). Maker and colleagues added Problem Types III and IV, to provide a more fluid transition between the Types, based on data observed in their research (C. June Maker, 1993; 1978; 1981; Whitmore & Maker, 1985). The DISCOVER Assessment and all DISCOVER Curriculum instruments use all of the Problem Types.
Figure 1: DISCOVER Problem Continuum

Problem
Type Examples
Type I: The problem and the method of solution are known to the problem presenter and the problem solver; the presenter knows the (one) correct solution. Solving math problems by a known algorithm or method; following a formula, in language, music, math or science; and performing prescribed body movements, as in dance or sports are Type I problems.
Type II: In Type II problems, the problem is known by the presenter and the solver, but the method of solution and solution are known only to the presenter. Type II is close to Type I in structure, except that the problem solver does not know the method by which to arrive at a solution. Problems such as mathematical “story problems” requiring the solver to figure out and apply the appropriate method to solve the problem; answering questions about factual material; scientific “experiments” with prescribed materials and variables; playing an instrument while sight reading the music; and creating a scale drawing are Type II problems.
Type III: The problem is known to the presenter and the solver, but more than one method may be used to arrive at the correct solution, which the presenter knows. Type III problems require a specific solution but many methods may be used to reach this solution. Finding the “key” to mathematical, word, or linguistic patterns; movement sequences created to meet specific requirements; and constructions using specified materials and meeting given criteria are Type III tasks.
Type IV: The problem is known to the presenter and the solver, but the problem may be solved in more than one way and the presenter knows the range of solutions. Problems that can be solved inductively but that have an accepted range of answers, such as geometry problems that may be solved using manipulatives; creating as many equations as possible using three (provided) numbers and the operations of addition and subtraction; writing Haiku; and creating music or movement sequences within defined parameters are examples of Type IV problems.
Type V: The problem is known to the presenter and the solver, but the method and solution are unknown to both. Type V problems are clearly defined, but methods and solutions are open. Questions such as, “In what ways might you share the results of your survey?” define Type V problems, as do constructions using specific materials and meeting pre-set goals, (such as building a mousetrap vehicle); creating prose or poetry; making a self-sustainable terrarium or aquarium; writing lyrics to an existing melody; writing a melody for existing lyrics; and finding new ways to apply existing formulas. Future Problem Solving (FPS) is a special case of Type V. The problem is known to the presenter and the solvers, and the solvers are taught the CPS process to use in developing their solution, but the solution is unknown to all.
Type VI: The problem is unknown or undefined and the method and solution are unknown to both presenter and solver. Type VI problems have the least structure; are the most complex; need to be defined and, possibly, redefined; and have numerous possible solutions. These are the problem situations we find in real-life that can be defined in more than one way and that may need redefining during the problem solving process. Type VI problem situations include those such as environmental pollution; student behavior; ethical behavior and standards; global warming; urban problems; social issues, such as violence or declining literacy; and international border issues. Type VI problems are not only appropriate for gifted students, but are a critical aspect of their education.
DISCOVER researchers are convinced that problem solving should play a key role in education, at all levels, precisely because it is so important in everyday life. Each of us may make hundreds of decisions every day, and the vast majority of these decisions fall in the range between a Type 3 and a Type 6, with most being closer to a Type 5 or 6. This fact is surprising to many people, until they think about it. Most decisions we make are not simple or closed because there are numerous possible variations or alternatives and there are myriad intended (or unintended) consequences to be considered. Getting dressed, for example, typically is a Type 4 problem; usually there is a clear need to do so, yet the method and solution will vary according to one’s state of mind, habit, the weather, the day’s planned events, and so forth. Getting dressed becomes a Type 5 problem on days when you are sick, or do not have specific plans, and you begin the day by asking, “Do I really need to get dressed or should I just stay in bed?”
DISCOVER was created because of a simple realization…that if real-life problems typically are in the Type 5 and Type 6 range, does it not make sense that we should test students’ ability to make these kinds of decisions? Likewise is it not imperative for educators to actually help students develop their problem solving skills, especially regarding complex, open-ended problems? Unfortunately much remains to be done in the standard classroom. Virtually all traditional testing methods, and most traditional lesson plans, continue to be designed around Problem Types 1 and 2 only—drill and practice, find-the-right-answer kinds of approaches. One individual observed that we spend twelve-plus years teaching our students how to solve Problem Types 1 and 2, and then expect them to function in the real world where these Problem Types are virtually non-existent.
Programs for gifted students usually are better, and are more likely to include all of the Problem Types. But even here a bizarre and little known situation occurs that can adversely affect a child’s development. The scenario is as follows: In most schools, superior performance on traditional, standardized tests scores is the predominant selection criterion used in programs for the gifted. Therefore because the questions on these tests contain only Problem Types 1 and 2, students who solve Problem Types 1 and 2 best are chosen. Then they are thrown into a track that emphasizes, instead, Problem Types 3, 4, 5, and 6, causing many to feel lost and frustrated. At the same time, those students who did not do as well on the standardized tests, perhaps because they are more comfortable with, and better at, solving Problem Types 3, 4, 5, and 6 are placed back in the standard curriculum that contains almost entirely Problem Types 1 and 2, causing them to feel frustrated and bored. DISCOVER researchers believe that the only way to remedy this mismatch is to determine which individuals can solve which Problem Types best (and in which areas), so that the curriculum is developmentally appropriate.
For those curious about how commonly used assessment methods and programs stack up, in terms of which Problem Types they include, see Figure 2 below.
Figure 2: Common Problem Types
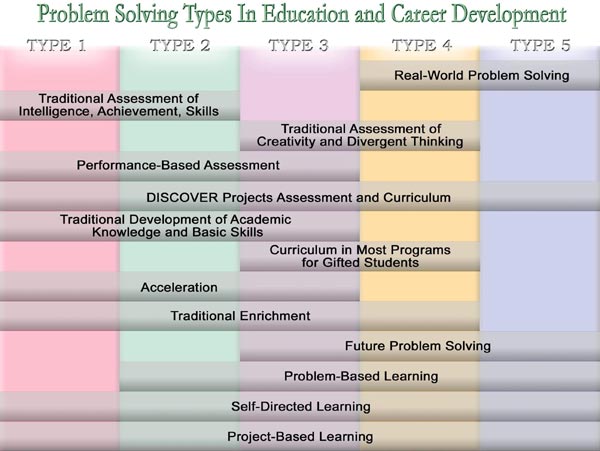
We acknowledge that changing the overall situation is complicated and will take time. It will require a systematic refocusing of perspective, such that testing and teaching methods begin to emulate real-world problem solving. We realize, too, that inclusion of Type 4 and Type 5 problems in testing and teaching is viewed as problematic because of the extra time required and the difficulty of “grading” such products. But we as educators must begin to ask tough questions. Are we adequately supplying our students with the skills they need to become successful adults? Are we testing what needs to be tested?
Some studies suggest, no. Scholars who have completed critical reviews of research (Baird, 1985; Hoyt, 1966; Nelson, 1975; O’Leary, 1980; Reilly & Chao, 1982; Wallach, 1976), including meta-analysis (Samson, et. al., 1984; Cohen, 1984), have concluded that although achievement and abilities test scores can predict future academic success (e.g. grades and later achievement test scores), their relationship to adult success factors is minimal. O’Leary (1980), for example, reports that the average correlation between measures of academic success and indicators of adult career success is .17. In other words, we are training our students how to pass tests but not necessarily how to succeed in life. Furthermore, reviews of research on problem solving (Rogers, 1993) have revealed that very few studies have ever included Type IV or V problem situations. As a result, little has been learned about the abilities and skills actually needed in the structuring and solving of real-world problems. Is it any surprise that teachers often are not sure how to teach the skills?
But progress is being made, both within DISCOVER and without. Tests of creativity, for example do tend to include more emphasis on complex, open-ended problems, and research on the predictive validity of these instruments is more encouraging. Only a few studies are available; the most notable is Torrance’s twenty-two-year follow-up of 211 individuals whose mean age was 27.5 years. All had been assessed with the Torrance Tests of Creative Thinking (TTCT) as elementary school students. Using a creativity index made up of scores on the TTCT over a three year period, and a variety of indicators of adult creative achievements, he found a correlation of .58 (p<.001) (Torrance, 1981). In a well designed reanalysis of Torrance’s data, Yamada and Tam (1996) found the four predictors—the creativity index, childhood future career image, IQ, and the presence of a mentor—explained 49% of the variance in adult creative achievement. The standardized regression coefficients were .44, .24, .20, and .16 respectively. The results of Torrance’s research are more conclusive than others, but the trend can be seen in numerous studies (c.f. Howieson, 1982; Baird, 1985).
In general, reviewers and authors conclude that the more the predictor (e.g. test or behavior such as high school or college achievements) resembles the criterion to be predicted (e.g. another test, grades, behaviors such as adult achievement), the more successful the prediction. For example, measures of creative writing predict later publications of fiction better than measures of general ability; the advanced knowledge sections of the GRE predict productivity in physics, geology, engineering and chemistry better than general verbal and quantitative sections; and student achievements in high school (e.g. publications, winning science fair competitions) predict later accomplishments—better than grades, academic achievement, or ability tests.
If we are to realize the vision articulated in the new curriculum standards and prepare a generation capable of designing new approaches to new problems, we must assess and develop a wide range of problem solving abilities. Instead of measuring and developing “decontextualized” knowledge or divergent thinking, we must measure the production, flexible use, originality, and addition of richness and detail in the solving of complex, realistic problems in specific academic and intellectual domains. This is the intent and promise of the DISCOVER assessment. We also must develop those abilities in real-life problem solving situations. This is the intent and promise of the DISCOVER curriculum.
References
Baird, L. L. (1985). Do grades and tests predict adult accomplishment? Research in Higher Education,23(1),3-85.
Cohen, P. A. (1984). College grades and adult achievement: A research synthesis. Research in Higher Education,20, 281-294.
Gallagher, S. A., & Rosenthal, H. (1992). The effects of problem-based learning on problem solving. Gifted Child Quarterly, 36(4), 195-200.
Getzels,J. & Csikszentmihalyi, M. (1967). Scientific creativity. Science Journal,3(9), 80-84.
Getzels, J. & Csikszentmihalyi, M. (1976). The creative vision: A longitudinal study of problem finding in art. New York: Wiley & Sons.
Howieson, H. (1982). A longitudinal study of creativity–1965–1975. The Journal of Creative Behavior,15(2), 117-135.
Hoyt, D. P. (1966). The relationship between college grades and adult achievement: A review of the literature. The Educational Record, 47, 70-75. (ACT Research Report 7. Iowa City, IA: American College Testing Program, 1965).
Maker, C.J. (1978). Successful Handicapped Adults. Unpublished doctoral dissertation. The University of Virginia.
Maker, C.J. (1981). The gifted hearing-impaired student. American Annals of the Deaf, 126, 631-645.
Maker, C.J. (1993). Creativity, intelligence, and problem solving: A definition and design for cross-cultural research and measurement related to giftedness. Gifted Education International,9(2), 68-77.
Maker, C.J. & Nielson, A.B. (1995). Teaching models in education of the gifted. 2nd Ed. Austin, TX: Pro-Ed.
Maker, C.J. & Schiever, S. (1991). Enrichment and acceleration: An overview and new directions. In G. Davis and N. Colangelo (Eds.) Handbook of Gifted Education. (pp. 99-110) Boston: Allyn & Bacon.
Nelson, A. M. (1975). Undergraduate academic achievement as an indicator of success. Washington, DC: Civil Service Commission.
O’Leary, B. S. (1980). College grade point average as an indicator of occupational success: An update (Personnel Research Report, 80, 281-294.
Reilly, R. R. & Chao, G. R. (1982). Validity and fairness of some alternative employee selection procedures. Personnel Psychology,35, 1-62.
Rogers, J.A. (1993) Understanding spatial intelligence through problem solving in art: An analysis of behavior, processes and products. Unpublished doctoral dissertation, The University of Arizona, Tucson.
Samson, G. R., Grave, M. E., Weinstein, T. & Walberg, H. J. (1984). Academic and occupational performance: a quantitative synthesis. American Educational Research Journal, 21, 311-322.
Torrance, E. P. (1981). Predicting the creativity of elementary school children. Gifted Child Quarterly, 25, 55-62.
Wallach, M. A. (1976). Tests tell us little about talent. American Scientist,64, January – February, 57-63.
Whitmore, J.R., & Maker, C.J. (1985). Intellectual giftedness in disabled persons. Austin, TX: Pro-Ed.
Yamada, H. & Tam, A. Y. (1996). Prediction study of adult creative achievement: Torrance’s longitudinal study revisited. Journal of Creative Behavior, 30 (2), 144-149.
